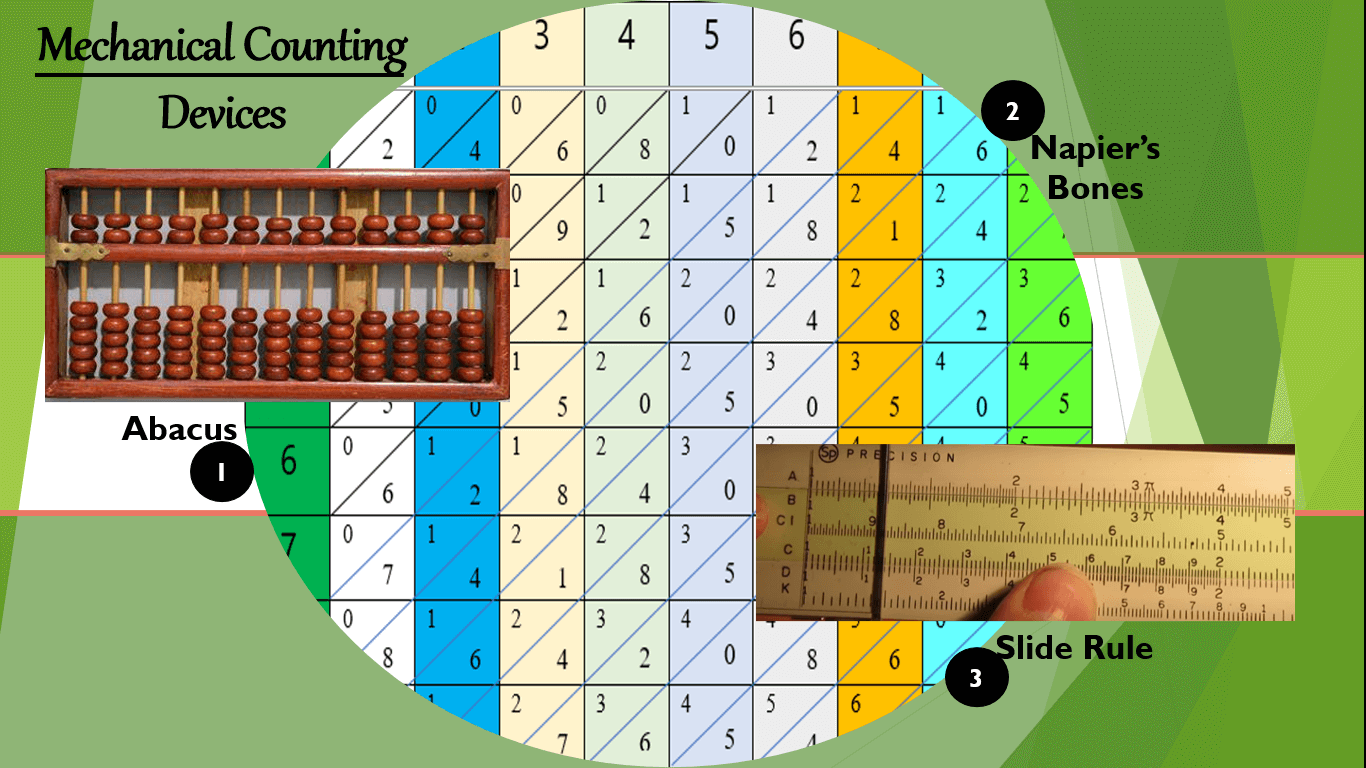
Mechanical counting devices are simple machines used to perform arithmetic operations. These machines are operated manually to perform basic calculations. Two major reasons led to the invention of these calculating devices:

1. The disadvantages of early counting devices
2. Increasing commercial activities

The problems associated with the early counting devices majorly led to the invention of simple tools that aid calculations. The number limitation and bulky nature of these devices made it difficult to compute complex numbers.

As cities began to develop, commercial activities and business transactions also began to grow. This growth led to complex additions, subtractions, and divisions that early counting devices cannot handle.

Also, there was growth in inter-city and inter-state business transactions making it difficult to carry bulky counting devices in transit. All these led to the invention of simple machines to replace the earlier devices. These newly found devices were later called mechanical calculating devices.

In this tutorial, you learn the following:

• What are mechanical counting devices?
• Examples of mechanical counting devices.

What is mechanical counting devices?

Mechanical counting devices are manually operated devices for counting and calculating numbers. They are simple equipment that can be operated manually by individuals.

Some of the equipment can be used to perform simple arithmetic operations like addition, subtraction, division, and multiplication.

Examples of Mechanical Counting Devices

There are three (3) main examples of mechanical counting devices. They include the following.

• Abacus
• The slide rule, and
• Napier’s bones.

Let us discuss each of them in detail.

Abacus

Abacus was one of the first calculating devices developed to help in arithmetic operations when trading between countries became important. Its nature and usage helped to overcome some of the problems associated with the early counting devices.

The abacus is made of beads or stones and strings or wires with marked-out positions. The position of a bead is used to determine its value. Four beads are in the down columns, and one bead is in the top columns. The bead in the top column is used to represent a 5. For example, to represent a seven, you will pull a top bead down and pull two bottom beads up.

In the beginning, the abacus was just a board with stones or sticks. On the surface of the abacus, there were parallel notches or grooves.

People made calculations by moving stones or sticks. If numbers are to be added, stones were added, if subtraction is to be made, stones were taken off. If it were multiplication, double summing was made. When dividing, double subtraction was performed.

The abacus counting device is an instrument used for counting as far back as 300 – 500 B.C. Its main objective is to make calculations easier and to suit the various number systems.

There are different types of abacus counting devices. They include the Chinese abacus called Suanpan which is still in use today. This device was invented in the 6^th century.

Also is the Roman abacus, named Calculi or Abaculi, and the Japanese abacus, called Soroban. Soroban was used in the 16th and 17th centuries.

As civilization advanced, the Chinese substituted stones for pearls and bullets and these were put into wire or string. Similarly, the Roman abacus was made of bronze, stone, ebony, or colored glass.

Napier’s bones

The abacus helped so much in performing additions and subtractions, but could not be used in complex multiplications. Then comes the invention of Napier’s bones.

Napier’s bones are rods on which numbers are marked. Each bone contains the multiplication of numbers 0 to 9. The value of the product of each number is written in a diagonal form as shown in the figure below.

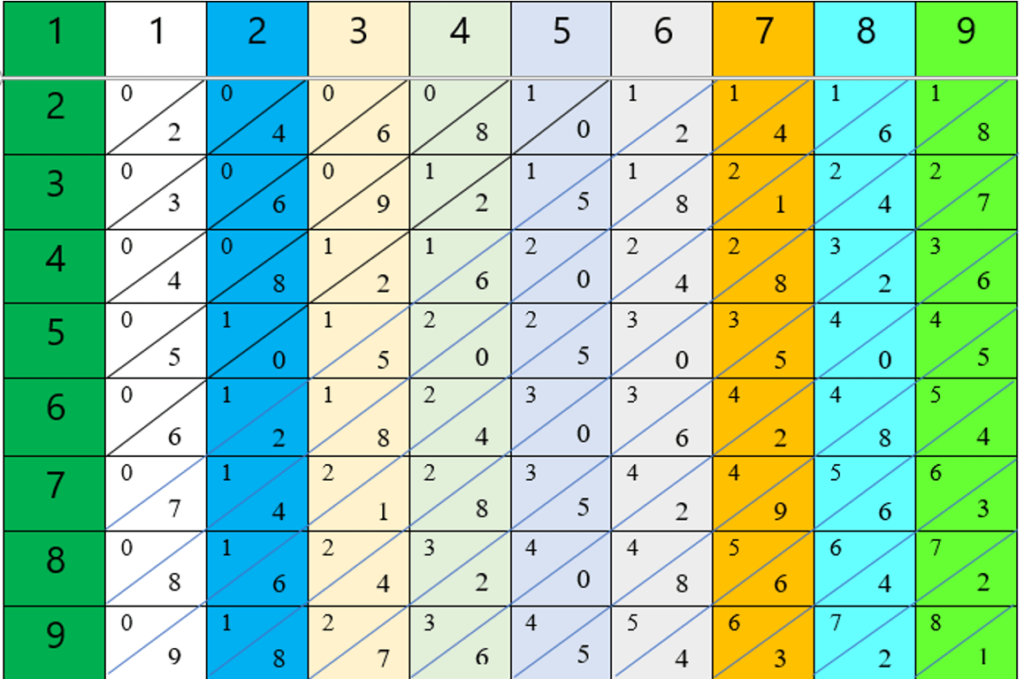
Note: I removed the zero (0) column since it is practically insignificant. Students can use a cardboard sheet, cut out 10 columns and mark them as shown in the figure above.

Multiply numbers

To perform simple multiplication, you will place the number to be multiplied along the first column. To perform complex multiplication, you place the numbers to be multiplied along the first column and then add the results.

For example, let us multiply the following numbers:

1. 258 x 5
2. 455 x 36

To perform the first multiplication, students should line up columns 2, 5, and 8 along the first column. Then add the values along the 5^th row as shown below.

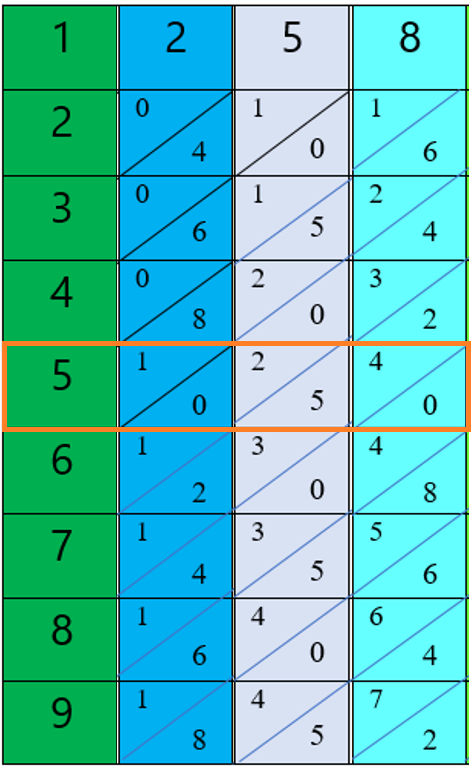
Do the following:

1. The first entry in column 2 is 1, record 1.
2. The second entry in column 2 is 0. Add the second entry to the first entry in column 5. You have [0 + 2 = 2]. Write down 2. Now you have 12.
3. The second entry in column 5 is 5. Add 5 to the first entry in column 8. You have [5 + 4 = 9]. You now have 129.
4. The second entry in column 8 is 0. Include 0 in your numbers to have: 1290. Therefore, 258 x 5 = 1290.

Note the following: if the added number exceeds 9, add the tenth unit value to the most left-hand value. For example, if we multiplied the 258 by 9, you will have:

1. 1.
2. [8 + 4 = 12]. Write down 2 and add 1 to the previous 1. You will have 22.
3. [5 + 7 = 12]. Write down 2 and add 1 to the previous 2. You will have 232.
4. Bring down 2 to finally have: 2322. Thus, 258 x 9 = 2322.

In the second example, do the following:

Lay columns 4,5, and 5 together with the first column, and extract rows 3 and 6.

1. Using the method discussed above, add column 3 to have: [1, 3, 6, and 5 = 1365]
2. Also, add column 6 to have: [2, 7, 3, and 0 = 2730]
3. Add the two results together starting with the last row (i.e. row 6): 1365
4. Move the first row one digit to the left and add 0 at the end as follow: 2730 + 13650 = 16380. Therefore, 455 x 36 = 16,380.

Divide numbers

The division is performed by repeated subtraction. For example, to divide 5768 by 25, do the following:

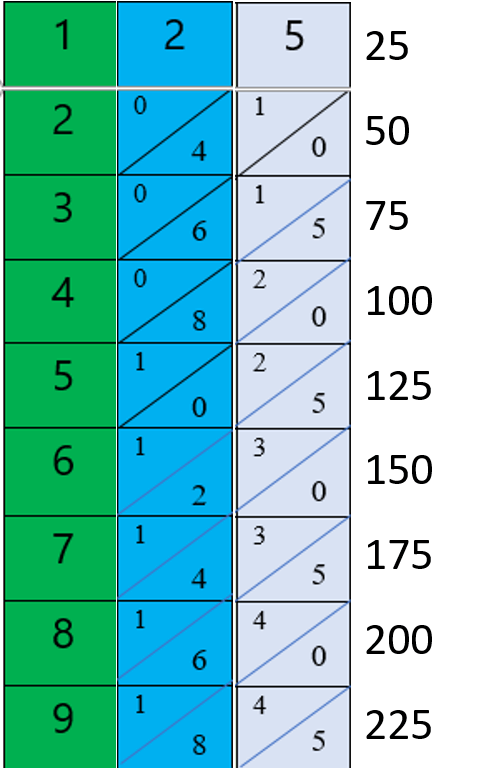
1. Lay columns 2 and 5 together with the first column, and obtain their corresponding products.
2. Locate the highest product value that is less than the first two digits in 5768. Of the products, 50 is the highest which is less than 57.
3. Subtract 50 from 57, and bring down the remainder. Then, write out the row that produced 50, which is row 2.
4. Bring down the next value (6) to have 76, and find the largest product that is less than 76. The value is 75. Write out the row value, that is 3.
5. Subtract 75 from 76 and bring down the remainder (1). Bring down the remaining value (8) to have 18.
6. Find the largest product that is less than 18. The value is 0. Write out the row value, which is 0.
7. The remainder of 18 is indivisible.

Therefore, 5768/25 = 230 18/25 = 230.72

Napier’s bones were invented in 1617 by a Scottish mathematician named John Napier. It is widely used to perform simple and complex multiplications and divisions. In some cases, it can be used to obtain square roots.

Napier later invented tables of Logarithms which enabled multiplication and division to be carried out by simple addition and subtraction.

Slide rule

The slide rule is an advanced manual device used to perform complex mathematical calculations. With the slide rule, you can perform multiplication, division, power, square root, and trigonometric functions calculations.

The slide rule is made up of two fixed bars and a center sliding bar. The metal window has a hairline feature that is called the cursor which is used for an accurate reading.

On the left-hand side of the slide rule are marked scales A – D. The number of scales on a slide rule varies depending on the number of mathematical functions the slide rule can perform.

Use the scales in A and B to perform multiplication and division and scales C and D for square and square roots. These numbers are marked according to a logarithmic scale with the first number on the slide rule scale being 1. This is because the log of zero is 1.

The slide rule was invented by an English mathematician and Anglican clergy, William Oughtred in 1622. This invention was necessitated by the invention of logarithms by John Napier. And the creation of logarithmic scales by Edmund Gunter.

This device is equivalent to today’s pocket calculator. To learn how to use the slide rule, visit the following resources: 2.972 How A Slide Rule Works (mit.edu).

Conclusion

The mechanical calculating devices are still useful because they help in teaching children basic calculations. With Napier’s bones children can learn how to perform multiplications and divisions using repeated additions and subtractions. The use of the abacus, Napier’s bones, and slide rule metamorphosed into the invention of electro-mechanical counting devices.