We use proportions to compare two numbers. For example, if the proportion of two numbers is increasing or decreasing in the same ratio, it is a direct proportionality.
Proportionality or proportion of two numbers is therefore a technique of comparing two numbers in order. The proportion calculator can be used to solve such equations.
The proportionality is denoted by the symbol “::” or by the “=”. The proportionality number is used to represent the equality or the relation of the two quantities.
We have heard the word proportionality when we are solving various problems in math. The ratio or the proportionality are the alternatives to each other. Solving proportions can be an uphill task if you cannot identify the direct and indirect proportionality.
In the following article, we are discussing the use of proportionality and ratio in various applications. We shall solve the direct and indirect proportionality of two quantities.
The proportion calculator can be of aid, especially, when computing direct proportions. In the end, you will learn how to solve the proportions of the direct and the indirect quantities.
Table of Contents
What is Proportion of two numbers?
Proportion can be defined as the ratio or comparative relationship between two numbers. When two sets of numbers are increasing or decreasing in the same ratio, we call it a direct proportion. Inversely, when one set is increasing and another is decreasing, we call it indirect proportion.
It is sometimes difficult to calculate the proportionality of two quantities manually. But through the help of the proportions calculator by calculator-online.net it can be simplified.
The proportions calculator makes it simple to identify the direct or indirect relationship between the two quantities. Proportionality can be applied in various situations. Let’s take a look at the different scenarios.
Direct proportionality
If two quantities, X and Y are increasing or decreasing together, it is called direct proportion.
Symbolically, we can say that: X = Y
For example, in a production environment, it takes more raw materials to produce more quantities and vice versa. Typically, we have a scenario like this:
Bolderer and Son’s Industries used 5kg of flour to produce 50 loaves of bread. How many kg of flour will be used to produce 200 loaves of bread?
This is a typical example of a direct proportion because producing more loaves requires more flour. How do we solve the above proportionality equation?
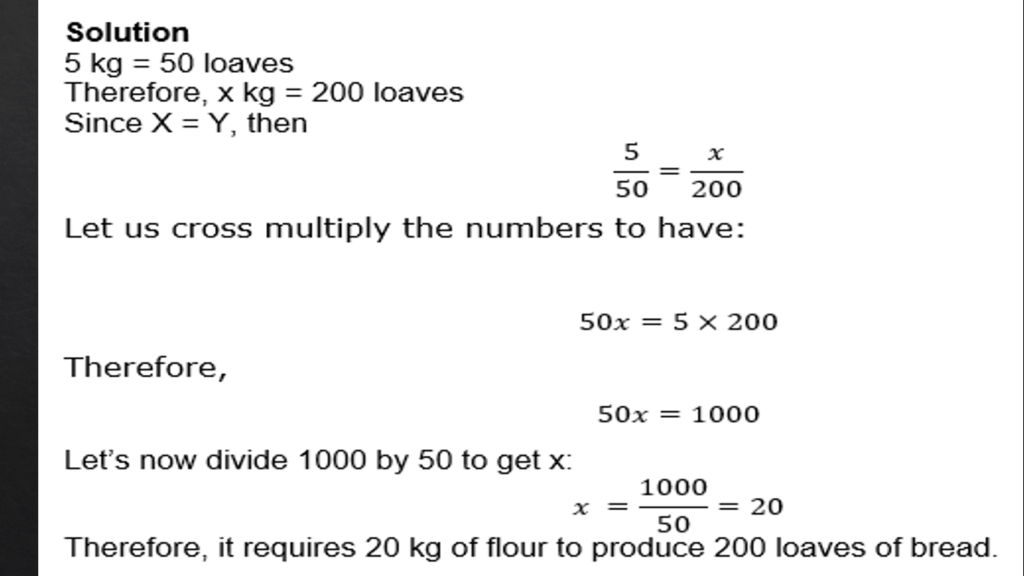
Solution
5 kg = 50 loaves
Therefore, x kg = 200 loaves
Since X = Y, then
Let us cross-multiply the numbers to have:
Therefore,
Let’s now divide 1000 by 50 to get x:
Therefore, it requires 20 kg of flour to produce 200 loaves of bread.
Another example of direct proportionality is speed and distance. Consider a vehicle that covered 200 km in one hour. If the speed of the vehicle is increased, then it will cover more kilometers in one hour.
When solving direct proportions always remember that a = b or x = y.
Indirect Proportionality
The difference between direct and indirect proportionality is the inverse relationship between two numbers. This is why it is also called inverse proportionality.
An inverse proportion of two numbers or quantities is such that one increases when the other decreases.
If quantity M is increasing, then quantity N decreasing.
Symbolically, we can say that: M = 1/N or M = c/N, where c = constant
When solving equations of this magnitude, you must be very careful not to make mistakes. But I will take you through some examples.
In a construction environment, for example, it takes more labor to complete a given task in fewer days and vice versa. E.g., if 20 laborers completed a task in 5 days, it should take fewer laborers to complete a similar task in 10 days.
Proportionality Examples
We have shown an example of direct proportion, which is quite easier to solve. In the following section, I will show you how to solve inverse proportions.
- The Setraco construction company discovered that it took 8 laborers 24 days to cast a 5km gutter in a waterway. They want to retain only 5 laborers to complete the remaining 5km. How many days will it take these 5 laborers to finish the same work?
Solution
This is a case of inverse proportion because it should take more labor to complete similar work in fewer days. Conversely, to complete similar work by 5 labor should take more days.
Since we have identified the type of proportionality, let’s solve the problem.
8 labor = 24 days
Therefore, 5 labor = x days
In inverse proportion, X = 1/Y,
Therefore,
(Where c is a constant)
Therefore,
Given x = 5 labor, to find the number of days, we solve as follows:
Then we have:
Therefore,
Let’s now divide 192 by 5 to get y:
Therefore, it should take 5 laborers 38.5 days to complete the remaining 5KM.

- Consider a job that is completed by 10 men in 4 days, how many men will do the same work in 5 days?
Solution
This is a case of inverse proportion because it should take fewer men to complete similar work in 5 days.
10 men = 4 days
Therefore, x men = 5 days
In inverse proportion, X = 1/Y,
Therefore,
(Where c is a constant)
Therefore,
Given y = 5 days, to find the number of men, we solve as follows:
Then we have:
Therefore,
Therefore, it should take 8 men 5 days to complete the same job, all things being equal.

Most times, it is difficult to determine if a problem is a direct or indirect proportion. This is where technology comes in.
The online calculator can be used to identify the various proportionality. It can be used to solve the direct and indirect proportionality by the ratio method and by the proportion method.
Direct and indirect proportionality is usually used to find the increasing or decreasing trend in two terms.
Ratios and proportions are used in everyday life as well as by businesses. For example, a business may use proportions to determine the amount of sales that yields an equivalent profit.
Also, proportions can be used in factory task completions and operational management.
Conclusion
Proportionality is a simple way to identify the relationship between different quantities. You may be surprised to learn the relationship of the proportional ratios. It is quite essential to know all the variables in the proportionality as described in the above examples.
Because of its relevance, not just in solving mathematical problems, but in everyday life, it is essential you understand its calculations.
Now, it’s your turn to respond. What did you learn and how difficult do you see the proportionality calculations?
Let us know by using the comment box below.




